The lengths of the two legs of a right triangle $PQR$ are given. Triangle $PQR$ is similar to $STU$, where $P$ corresponds to $S$ and $Q$ corresponds to $T$. What is the value of $sin(U)$?
$$PQ=7$$
$$QR=24$$
Answer:
B
Explanation
The correct answer is Choice B.
Draw the triangle $PQR$ and find $sin(R)$ which is equal to $sin(U)$ due to similarity of triangle $STU$.
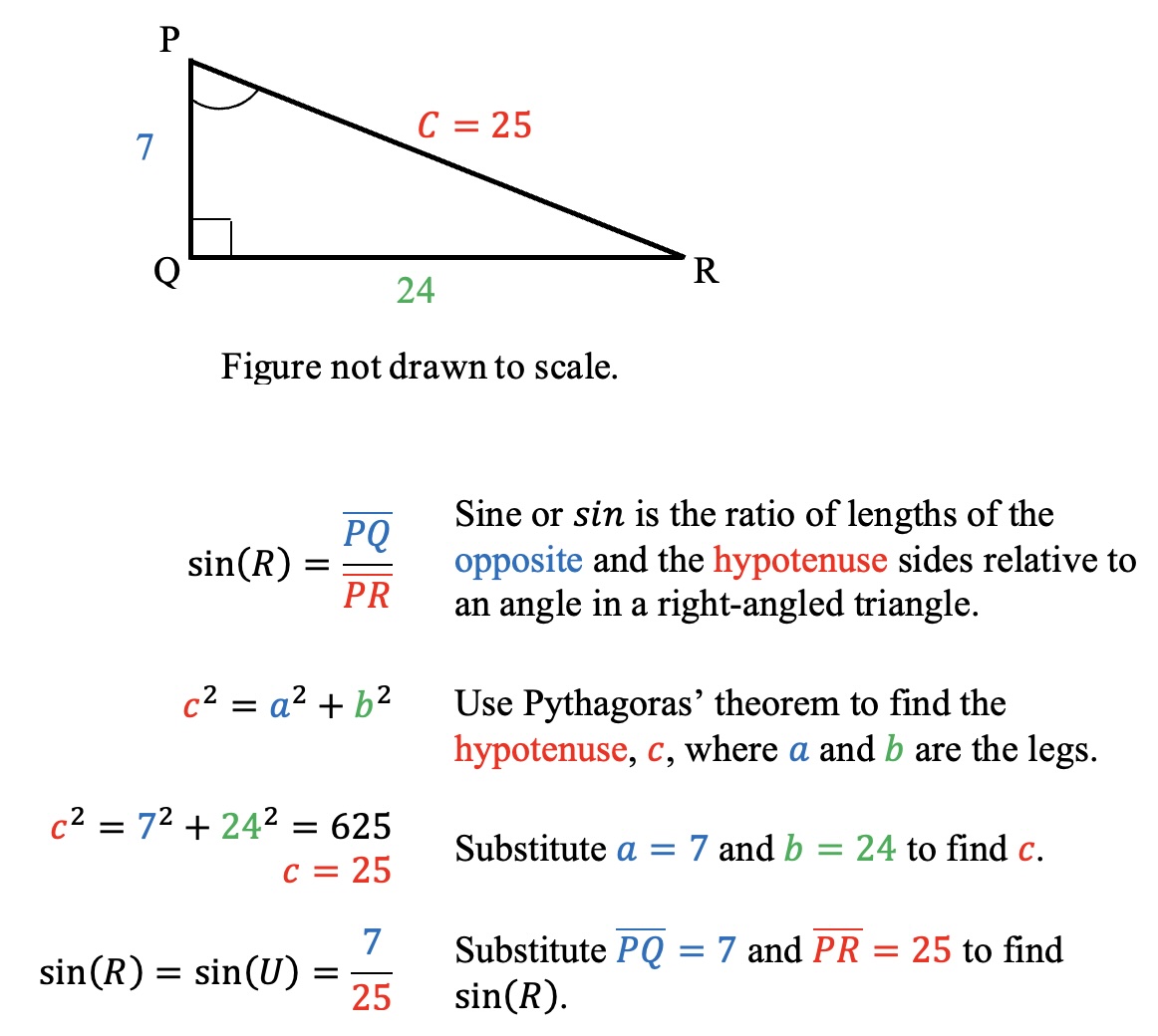
Choice A is incorrect and may result from finding $tan(U)$, instead of $sin(U)$.
Choice C is incorrect and may result from finding $cos(U)$, instead of $sin(U)$.
Choice D is incorrect and may result from finding $tan(S)$, instead of $sin(U)$.
✨ Expert's Tip ✨
The dimensions of similar triangles are not always the same, but the ratio of the side lengths is the same. Also, all the internal angles are identical.