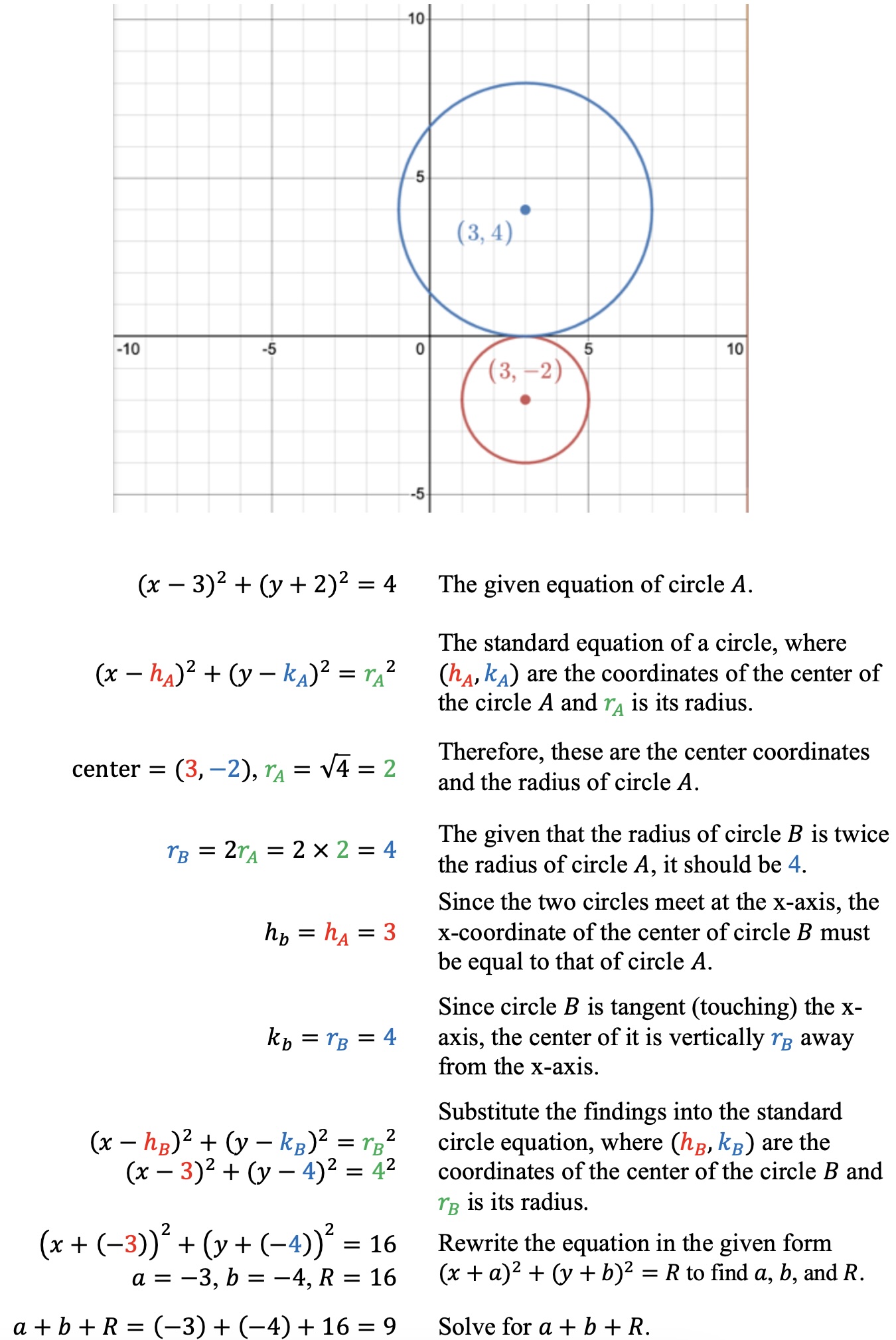
Math Module 2 (Hard) Question 9
Circle $A$ in the $xy$-plane has the equation $(x-3)^2+(y+2)^2=4$. Circle $B$ meets Circle $A$ at a point on the $x$-axis and has the radius that is twice the radius of Circle A. The equation defining Circle $B$ in the $xy$-plane is $(x+a)^2+(y+b)^2=R$, where $a$, $b$, and $R$ are constants. What is the value of $a+b+R$?